Suppose all firms in an industry have a production technology described by the production function 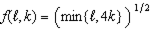 . The cost of labor is 2 and the cost of capital is 4, and each firm faces a recurring fixed cost of 300.
. The cost of labor is 2 and the cost of capital is 4, and each firm faces a recurring fixed cost of 300.
a. Derive the long run cost and average cost functions for each firm. (Hint: Given the shapes of the isoquants implied by the production function, you should be able to do this without solving a calculus problem.)
b. What is the long run equilibrium output price?
c. How much does each firm produce in long run equilibrium?
d. Suppose market demand is given by 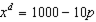
e. Suppose the industry is currently in long run equilibrium. Derive the short run cost function for each firm (assuming labor is variable but capital is fixed in the short run).
f. Now suppose that demand falls to 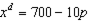
What will be an ideal response?
 units of labor and
units of labor and 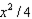 unit of capital. Thus, the cost of the inputs for x units of output is given by
unit of capital. Thus, the cost of the inputs for x units of output is given by 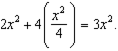 Including the recurring fixed cost, we then get a cost function of
Including the recurring fixed cost, we then get a cost function of  This implies an average cost function of
This implies an average cost function of 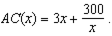
b. The long run output price occurs at the lowest point of the firms' AC curve -- i.e. where the derivative of AC(x) is zero. Solving
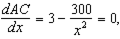 we get x=10, and plugging this back into the AC function, we get a long run equilibrium price of 60.
we get x=10, and plugging this back into the AC function, we get a long run equilibrium price of 60.c. We just calculated in (b) that each firm produces 10 units of output in long run equilibrium.
d. At the long run equilibrium price of 60, the quantity demanded is 400. With each firm producing 10, this implies 40 firms in the industry.
e. Since each firm is producing 10 in the LR equilibrium, each firm is using 100 units of labor and 25 units of capital. In the short run, firms can't produce more than 10 units of output (because of the extreme complementarity of inputs in production), but it can produce less by hiring less labor. The short run cost function for output is then

f. The short run market supply curve is the sum of the short run MC curves. We just calculated the short run cost curve for each firm -- and from that we can derive the MC curve as
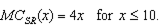 Setting MC equal to p, we can write the firm supply function as x(p)=p/4 -- and adding that over 40 firms, we get the short run market supply function of X(p)=10p. But, with 40 firms and each unable to produce more than 10 units in the short run, this implies the market supply function is
Setting MC equal to p, we can write the firm supply function as x(p)=p/4 -- and adding that over 40 firms, we get the short run market supply function of X(p)=10p. But, with 40 firms and each unable to produce more than 10 units in the short run, this implies the market supply function is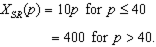
Setting the new demand function equal to this supply function, we get p=35.
In the long run, price has to go back up to 60 (since firm costs have not changed) -- at which price only 100 units are demanded. This implies the number of firms will drop from 40 to 10.
You might also like to view...
Workers and firms both expect that prices will be 3% higher next year than they are this year. As a result,
A) the short-run aggregate supply curve will shift to the left as wages increase. B) the purchasing power of wages will rise if wages increase by 3%. C) workers will be willing to take lower wages next year. D) aggregate demand will increase by 3%.
One of the objectives of supply-side policies is to
a. focus attention on the trade-off between inflation and unemployment. b. sharpen the trade-off between inflation and unemployment. c. eliminate the trade-off between inflation and unemployment. d. convince the public of the trade-off between inflation and unemployment.